
To apply this test, a continuous normally distributed variable (Test variable) and a categorical variable with two categories (Grouping variable) are used.
The independent t test, also called unpaired t test, is an inferential statistical test that determines whether there is a statistically significant difference between the means in two unrelated (independent) groups? One sample t test indicated that mean difference between sample mean and population mean was statistically significantly different to each other ( P = 0.045).

Įxample: From Table 1, BMI (mean ± SD) was given 24.45 ± 2.19, whereas population mean was assumed to be 25.5.
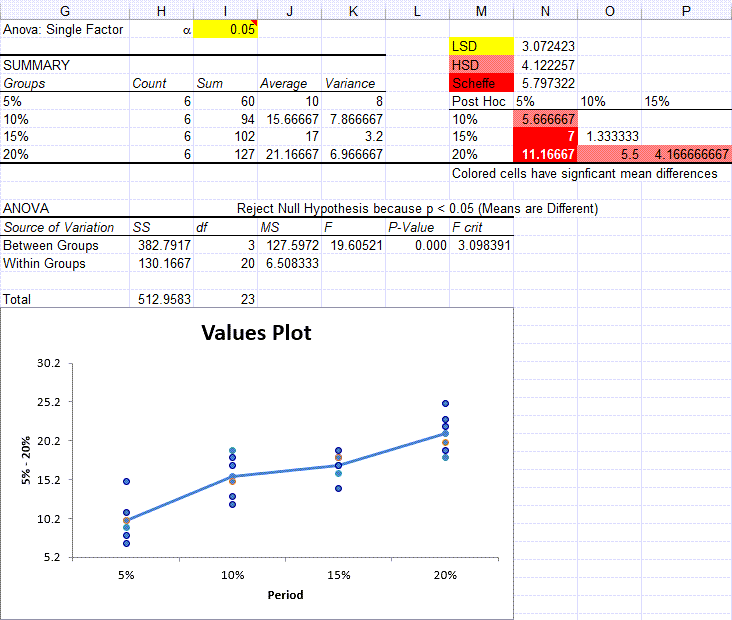
#Survey data to use for two way anova in excel software
To apply this test through popular statistical software i.e., statistical package for social sciences (SPSS), option can be found in the following menu. In one sample Z test, tabulated value is z value (instead of t value in one sample t test). If population SD is not known, one sample t test can be used at any sample size. In case sample size is ≥30 used to prefer one sample z test over one sample t test although for one sample z test, population SD must be known. One-sample t test is used when sample size is <30. Sample should be continuous variable and normally distributed. To apply this test, mean, standard deviation (SD), size of the sample (Test variable), and population mean or hypothetical mean value (Test value) are used. The one sample t test is a statistical procedure used to determine whether mean value of a sample is statistically same or different with mean value of its parent population from which sample was drawn. Further, examples related to the above statistical methods are discussed from the given data. To understand the above statistical methods, an example with a data set of 20 patients whose age groups, gender, body mass index (BMI), and diastolic blood pressure (DBP) measured at baseline (B/L), 30 min and 60 min are given below. The aim of the present article is to discuss the assumptions, application, and interpretation of the some popular T, ANOVA, and ANCOVA methods i.e., one sample t test, independent samples t test, paired samples t test, one-way ANOVA, two-ways ANOVA, one-way repeated measures ANOVA, two-ways repeated measures ANOVA, one-way ANCOVA, and One-way repeated measures ANCOVA. Although not every method is popular, some of them can be managed from other available methods. There are many statistical tests within Student's t test ( t test), ANOVA and ANCOVA, and each test has its own assumptions.
Most parametric test has an alternative nonparametric test. For non-normal continuous variable, median is representative measure, and in this situation, comparison between the groups is performed using non-parametric methods. Mean is the representative measure for normally distributed continuous variable and statistical methods used to compare between the means are called parametric methods. For these methods, testing variable (dependent variable) should be in continuous scale and approximate normally distributed. Student's t test ( t test), analysis of variance (ANOVA), and analysis of covariance (ANCOVA) are statistical methods used in the testing of hypothesis for comparison of means between the groups.


 0 kommentar(er)
0 kommentar(er)
